Page 288 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

Notes
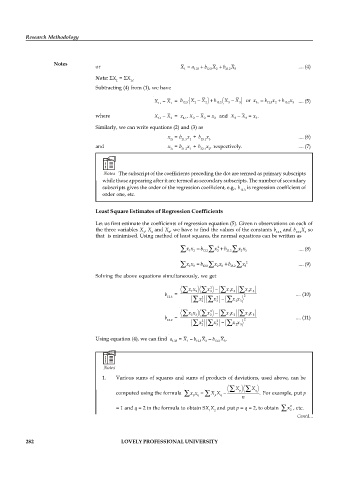
or X = a + b X + b X .... (4)
1 1.23 12.3 2 13.2 3
Note: X = X .
1 1c
Subtracting (4) from (1), we have
X - X = b (X - X ) b+ (X - X ) or x = b x + b x 3 .... (5)
1c 1 12.3 2 2 13.2 3 3 1c 12.3 2 13.2

where X - X = x , X - X = x and X - X = x .
1c 1 1c 2 2 2 3 3 3
Similarly, we can write equations (2) and (3) as
x = b x + b x .... (6)
2c 21.3 1 23.1 3
and x = b x + b x , respectively. .... (7)
3c 31.2 1 32.1 2

Notes The subscript of the coefficients preceding the dot are termed as primary subscripts
while those appearing after it are termed as secondary subscripts. The number of secondary
subscripts gives the order of the regression coefficient, e.g., b is regression coefficient of
12.3
order one, etc.

Least Square Estimates of Regression Coefficients
Let us first estimate the coefficients of regression equation (5). Given n observations on each of
the three variables X , X and X , we have to find the values of the constants b and b X so
1 2 3 12.3 13.2 3
that is minimised. Using method of least squares, the normal equations can be written as
å x x = b 12.3å x + b 13.2å x x 3 .... (8)
2
1 2
2
2
å x x = b 12.3å x x + b 13.2å x 2 3 .... (9)
1 3
3
2
Solving the above equations simultaneously, we get
(å x x )(å x - x x )(å x x )
) (å
2
b = 1 2 3 1 3 2 2 3 .... (10)
) (å
12.3 (å x 2 2 )(å x - x x )
2
2 3
3
) (å
(å x x )(å x - x x )(å x x )
2
b = 1 3 2 1 2 2 3 .... (11)
) (å
(å x 2 2 )(å x - x x
13.2 ) 2
2
2 3
3
Using equation (4), we can find a = X - b X - b X .
1.23 1 12.3 2 13.2 3
Notes
1. Various sums of squares and sums of products of deviations, used above, can be
(å X )(å X )
p q å
computed using the formula å x x = X X - p q . For example, put p
p
q
n
= 1 and q = 2 in the formula to obtain SX X and put p = q = 2, to obtain å x 2 2 , etc.
1 2
Contd...
282 LOVELY PROFESSIONAL UNIVERSITY