Page 217 - DCOM504_SECURITY_ANALYSIS_AND_PORTFOLIO_MANAGEMENT

Security Analysis and Portfolio Management

Notes perfect market will be statistically independent of one another. If stock price changes behave
like a series of results obtained by flipping a coin, does this mean that on average stock price
changes have zero mean? Not necessarily. Since stocks are risky, we actually expect to find a
positive mean change in stock prices.

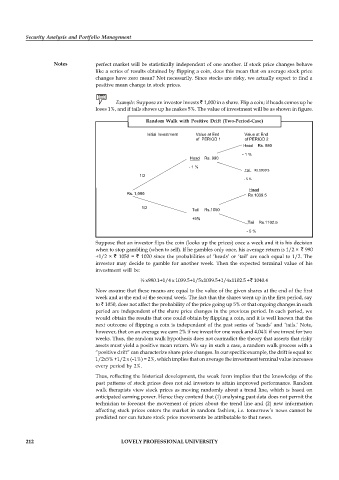
Example: Suppose an investor invests 1,000 in a share. Flip a coin; if heads comes up he
loses 1%, and if tails shows up he makes 5%. The value of investment will be as shown in figure.

Random Walk with Positive Drift (Two-Period-Case)

Initial Investment Value at End Value at End
of PERIOD 1 of PERIOD 2
Head Rs. 980

- 1 %
Head Rs. 990

- 1 %
Tail Rs.1039.5
1/2
- 5 %

Head
Rs. 1,000
Rs.1039.5

- 1 %
1/2 Tail Rs.1050

+5%
Tail Rs.1102.5

- 5 %

Suppose that an investor flips the coin (looks up the prices) once a week and it is his decision

when to stop gambling (when to sell). If he gambles only once, his average return is 1/2 × 990
+1/2 × 1050 = 1020 since the probabilities of ‘heads’ or ‘tail’ are each equal to 1/2. The
investor may decide to gamble for another week. Then the expected terminal value of his
investment will be:
½ x980.1+1/4 x 1039.5+1/5x1039.5+1/4x1102.5 + 1040.4
Now assume that these means are equal to the value of the given shares at the end of the first
week and at the end of the second week. The fact that the shares went up in the first period, say
to 1050, does not affect the probability of the price going up 5% or that ongoing changes in each
period are independent of the share price changes in the previous period. In each period, we
would obtain the results that one could obtain by flipping a coin, and it is well known that the
next outcome of flipping a coin is independent of the past series of ‘heads’ and ‘tails.’ Note,
however, that on an average we earn 2% if we invest for one week and 4.04% if we invest for two
weeks. Thus, the random walk hypothesis does not contradict the theory that asserts that risky
assets must yield a positive mean return. We say in such a case, a random walk process with a
“positive drift” can characterize share price changes. In our specific example, the drift is equal to:
1/2x5% +1/2 x (–1%) = 2%, which implies that on average the investment terminal value increases
every period by 2%.
Thus, reflecting the historical development, the weak form implies that the knowledge of the
past patterns of stock prices does not aid investors to attain improved performance. Random
walk therapists view stock prices as moving randomly about a trend line, which is based on
anticipated earning power. Hence they contend that (1) analysing past data does not permit the
technician to forecast the movement of prices about the trend line and (2) new information
affecting stock prices enters the market in random fashion, i.e. tomorrow’s news cannot be
predicted nor can future stock price movements be attributable to that news.

212 LOVELY PROFESSIONAL UNIVERSITY