Page 88 - DCOM504_SECURITY_ANALYSIS_AND_PORTFOLIO_MANAGEMENT

Unit 2: Risk and Return

When Diversification does not Help Notes

Perfectly Positively Correlated Returns
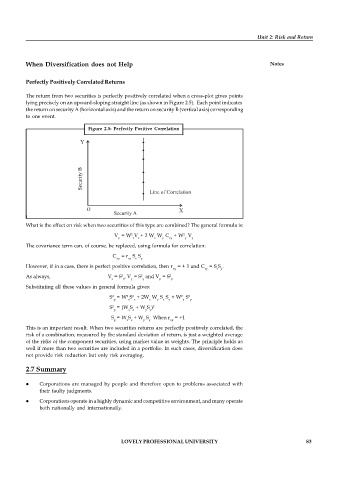
The return from two securities is perfectly positively correlated when a cross-plot gives points
lying precisely on an upward-sloping straight line (as shown in Figure 2.5). Each point indicates
the return on security A (horizontal axis) and the return on security B (vertical axis) corresponding
to one event.

Figure 2.5: Perfectly Positive Correlation

Y

Security B

Line of Correlation

O X
Security A

What is the effect on risk when two securities of this type are combined? The general formula is:
2
2
V = W V + 2 W W C + W V
p x x x y xy y y
The covariance term can, of course, be replaced, using formula for correlation:
C = r S S
xy xy x y
However, if in a case, there is perfect positive correlation, then r = + 1 and C = S S .
xy xy x y
2
2
As always, V = S , V = S and V = S 2
x x y y p p
Substituting all these values in general formula gives:
2
2
2
2
S = W S + 2W W S S + W S 2
p x x x y x y y y
S = (W S + W S ) 2
2
p x x y y
S = W S + W S When r = +1
p x x y y xy
This is an important result. When two securities returns are perfectly positively correlated, the
risk of a combination, measured by the standard deviation of return, is just a weighted average
of the risks of the component securities, using market value as weights. The principle holds as
well if more than two securities are included in a portfolio. In such cases, diversification does
not provide risk reduction but only risk averaging.
2.7 Summary

Corporations are managed by people and therefore open to problems associated with
their faulty judgments.

Corporations operate in a highly dynamic and competitive environment, and many operate
both nationally and internationally.

LOVELY PROFESSIONAL UNIVERSITY 83