Page 88 - DCOM507_STOCK_MARKET_OPERATIONS

Unit 4: Risk and Return

Notes
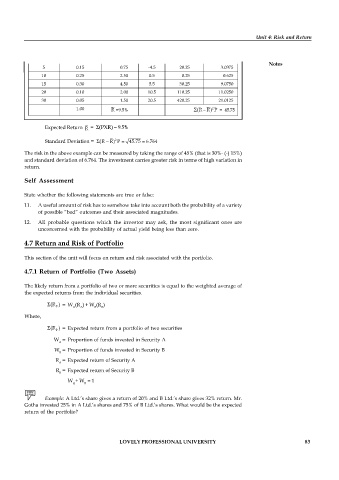
5 0.15 0.75 -4.5 20.25 3.0375
10 0.25 2.50 0.5 0.25 0.625
15 0.30 4.50 5.5 30.25 9.0750
20 0.10 2.00 10.5 110.25 11.0250
30 0.05 1.50 20.5 420.25 21.0125
2
1.00 R =9.5% Σ(R–R) P = 45.75

Expected Return R = (PXR) 9.5%Σ =

Standard Deviation = (R R) PΣ − 2 = 45.75 = 6.764
The risk in the above example can be measured by taking the range of 45% (that is 30%- (-) 15%)
and standard deviation of 6.764. The investment carries greater risk in terms of high variation in
return.

Self Assessment

State whether the following statements are true or false:
11. A useful amount of risk has to somehow take into account both the probability of a variety
of possible “bad” outcomes and their associated magnitudes.
12. All probable questions which the investor may ask, the most significant ones are
unconcerned with the probability of actual yield being less than zero.

4.7 Return and Risk of Portfolio

This section of the unit will focus on return and risk associated with the portfolio.

4.7.1 Return of Portfolio (Two Assets)

The likely return from a portfolio of two or more securities is equal to the weighted average of
the expected returns from the individual securities.
P =W (R ) + W (R )
Σ (R ) A A B B
Where,

P = Expected return from a portfolio of two securities
Σ (R )
W = Proportion of funds invested in Security A
A
W = Proportion of funds invested in Security B
B
R = Expected return of Security A
A
R = Expected return of Security B
B
W + W = 1
A B

Example: A Ltd.’s share gives a return of 20% and B Ltd.’s share gives 32% return. Mr.
Gotha invested 25% in A Ltd.’s shares and 75% of B Ltd.’s shares. What would be the expected
return of the portfolio?

LOVELY PROFESSIONAL UNIVERSITY 83