Page 180 - DMTH201_Basic Mathematics-1

Unit 6: Functions

t
We also know what 2 means if t = p/q is a rational number, the ratio of two integers, 2 p/q is the Notes
th
th
q root of the p power of 2.
2 1/2 = 2 = 1.4142
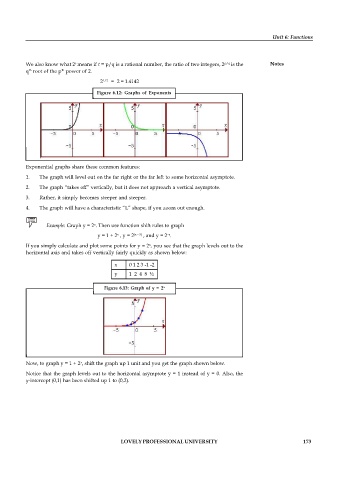
Figure 6.12: Graphs of Exponents

Exponential graphs share these common features:
1. The graph will level out on the far right or the far left to some horizontal asymptote.

2. The graph “takes off” vertically, but it does not approach a vertical asymptote.
3. Rather, it simply becomes steeper and steeper.
4. The graph will have a characteristic “L” shape, if you zoom out enough.

x
Example: Graph y = 2 . Then use function shift rules to graph
x
y = 1 + 2 , y = 2 (x – 3) , and y = 2 .
–x
x
If you simply calculate and plot some points for y = 2 , you see that the graph levels out to the
horizontal axis and takes off vertically fairly quickly as shown below:
x 0 1 2 3 -1 -2
y 1 2 4 8 ½

Figure 6.13: Graph of y = 2 x

x
Now, to graph y = 1 + 2 , shift the graph up 1 unit and you get the graph shown below.
Notice that the graph levels out to the horizontal asymptote y = 1 instead of y = 0. Also, the
y-intercept (0,1) has been shifted up 1 to (0,2).

LOVELY PROFESSIONAL UNIVERSITY 173