Page 222 - DMTH201_Basic Mathematics-1
P. 222
Unit 7: Limits
Notes
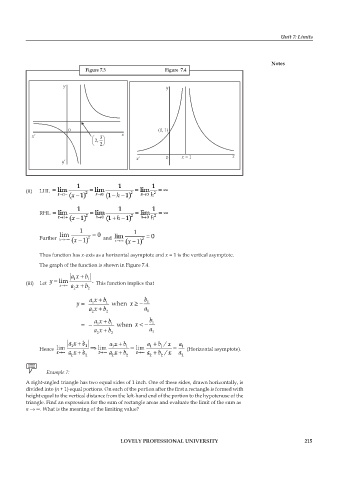
Figure 7.3 Figure 7.4
y y
0 (0, 1)
x′ 3 x
2,
2
x′ 0 x = 1 x
y′
(ii) LHL
RHL
Further and
Thus function has x-axis as a horizontal asymptote and x = 1 is the vertical asymptote.
The graph of the function is shown in Figure 7.4.
(iii) Let This function implies that
y = when
= when
Hence (Horizontal asymptote).
Example 7:
A right-angled triangle has two equal sides of 1 inch. One of these sides, drawn horizontally, is
divided into (n + 1) equal portions. On each of the portion after the first a rectangle is formed with
height equal to the vertical distance from the left-hand end of the portion to the hypotenuse of the
triangle. Find an expression for the sum of rectangle areas and evaluate the limit of the sum as
n → ∞. What is the meaning of the limiting value?
LOVELY PROFESSIONAL UNIVERSITY 215