Page 225 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

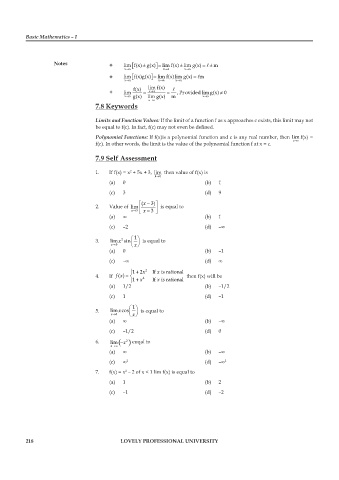
Notes ™ z lim ( ) g ( )˘= x lim ( ) lim ( ) = x ± l m
±
x ±
f È
x
g
f
x Æ a Î ˚ x Æ a xÆ a
z lim ( ) ( )˘= ™ Î f È x g x ˚ lim ( )lim ( ) = f x g x l m
x Æ a x Æ a xÆ a
f
f ( ) x lim ( ) x l
g
™ z lim = xÆ a = , Provided lim ( ) x π 0 0
g
xÆ a g ( ) x lim ( ) x m xÆ a
xÆ a
7.8 Keywords
Limits and Function Values: If the limit of a function f as x approaches c exists, this limit may not
be equal to f(c). In fact, f(c) may not even be defined.
Polynomial Functions: If f(x)is a polynomial function and c is any real number, then lim f(x) =
f(c). In other words, the limit is the value of the polynomial function f at x = c. x→ c
7.9 Self Assessment
1. If f(x) = x + 5x + 3, lim then value of f(x) is
2
h→ 0
(a) 0 (b) 1
(c) 3 (d) 9

2. Value of lim  (x − 3)  is equal to
x→ 3   x − 3 
(a) ∞ (b) 1
(c) –2 (d) –∞
1

3. limx 2 sin  is equal to
x
x→ 0 
(a) 0 (b) –1
(c) –∞ (d) ∞
x
1 2x
 + 2 If is rational
x
4. If f () =  4 then f(x) will be
+
x
 1 x If is rational
(a) 1/2 (b) –1/2
(c) 1 (d) –1
1

x
5. lim cos  is equal to
x
x→ 0 
(a) ∞ (b) –∞
(c) –1/2 (d) 0
( )
6. lim − x euqal to
2
x→∞
(a) ∞ (b) –∞
(c) ∞ 2 (d) –∞ 2
7. f(x) = x – 2 of x < 1 lim f(x) is equal to
2
(a) 1 (b) 2
(c) –1 (d) –2

218 LOVELY PROFESSIONAL UNIVERSITY