Page 229 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

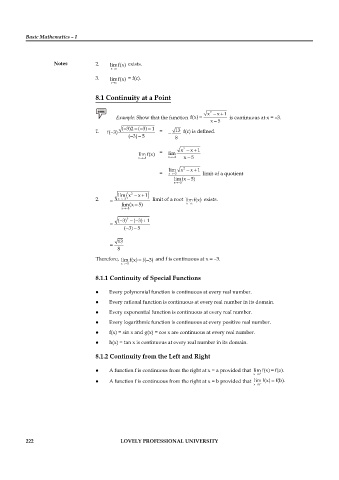
Notes 2. exists.

3. = f(c).

8.1 Continuity at a Point

Example: Show that the function is continuous at x = –3.

1. = f(c) is defined.

=

= limit of a quotient

2. limit of a root exists.

Therefore, and f is continuous at x = –3.

8.1.1 Continuity of Special Functions

Every polynomial function is continuous at every real number.
Every rational function is continuous at every real number in its domain.
Every exponential function is continuous at every real number.
Every logarithmic function is continuous at every positive real number.
f(x) = sin x and g(x) = cos x are continuous at every real number.

h(x) = tan x is continuous at every real number in its domain.

8.1.2 Continuity from the Left and Right

A function f is continuous from the right at x = a provided that
A function f is continuous from the right at x = b provided that

222 LOVELY PROFESSIONAL UNIVERSITY