Page 283 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
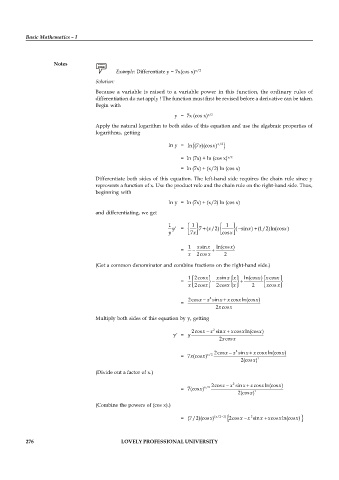
Example: Differentiate y = 7x(cos x) x/2
Solution:

Because a variable is raised to a variable power in this function, the ordinary rules of
differentiation do not apply ! The function must first be revised before a derivative can be taken.
Begin with
y = 7x (cos x) x/2
Apply the natural logarithm to both sides of this equation and use the algebraic properties of
logarithms, getting

ln y = ln (7 )(cos )x x x /2
= ln (7x) + ln (cos x) x/2
= ln (7x) + (x/2) ln (cos x)

Differentiate both sides of this equation. The left-hand side requires the chain rule since y
represents a function of x. Use the product rule and the chain rule on the right-hand side. Thus,
beginning with
ln y = ln (7x) + (x/2) ln (cos x)
and differentiating, we get

1 1 1
y = 7 ( /2) ( sin ) (1/2)ln(cos )
x
x
x
y 7x cosx
1 x sin x ln(cos )
x
=
x 2cosx 2
(Get a common denominator and combine fractions on the right-hand side.)
x
1 2cosx x sinx x ln(cos ) x cosx
=
x 2cosx 2cos x x 2 x cosx
2cosx x 2 sinx x cos ln(cos )
x
x
=
x
2 cosx
Multiply both sides of this equation by y, getting
x
x
2cosx x 2 sinx x cos ln(cos )
y = y
x
2 cosx
x
x
/2 2cosx x 2 sinx x cos ln(cos )
= 7 (cos )x x x 1
x
2(cos )
(Divide out a factor of x.)
x
x
/2 2cosx x 2 sinx x cos ln(cos )
= 7(cos )x x
2(cos ) 1
x
(Combine the powers of (cos x).)
x
x
= (7/2)(cos )x ( /2 1) 2cosx x 2 sinx x cos ln(cos )
x
276 LOVELY PROFESSIONAL UNIVERSITY