Page 333 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
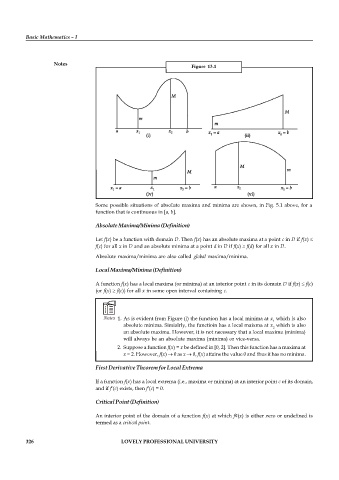
Figure 13.1

Some possible situations of absolute maxima and minima are shown, in Fig. 5.1 above, for a
function that is continuous in [a, b].

Absolute Maxima/Minima (Definition)

Let f(x) be a function with domain D. Then f(x) has an absolute maxima at a point c in D if f(x)
f(c) for all x in D and an absolute minima at a point d in D if f(x) f(d) for all x in D.
Absolute maxima/minima are also called global maxima/minima.

Local Maxima/Minima (Definition)

A function f(x) has a local maxima (or minima) at an interior point c in its domain D if f(x) f(c)
(or f(x) f(c)) for all x in some open interval containing c.

Notes 1. As is evident from Figure (i) the function has a local minima at x which is also
1
absolute minima. Simialrly, the functioin has a local maixma at x which is also
2
an absolute maxima. However, it is not necessary that a local maxima (minima)
will always be an absolute maxima (minima) or vice-versa.
2. Suppose a function f(x) = x be defined in [0, 2]. Then this function has a maxima at
x = 2. However, f(x) 0 as x 0, f(x) attains the value 0 and thus it has no minima.

First Derivative Theorem for Local Extrema

If a function f(x) has a local extrema (i.e., maxima or minima) at an interior point c of its domain,
and if f (c) exists, then f (c) = 0.

Critical Point (Definition)

An interior point of the domain of a function f(x) at which f¢(x) is either zero or undefined is
termed as a critical point.

326 LOVELY PROFESSIONAL UNIVERSITY