Page 112 - DMTH202_BASIC_MATHEMATICS_II

Unit 8: Formation of Differential Equation

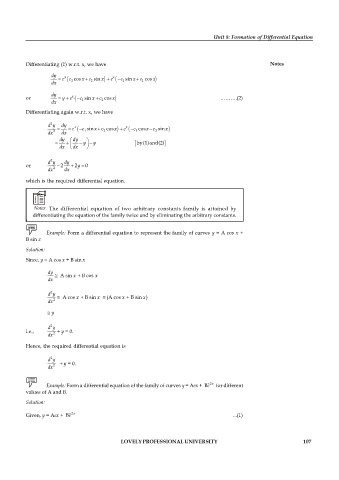
Differentiating (1) w.r.t. x, we have Notes
dy x x
 e c cosx  c 2 sinx  e  sinc 1 x c 2 cosx 
 1
dx
dy
or   e x  sinc 1 x  c 2 cosx  ……….(2)
y
dx
Differentiating again w.r.t. x, we have

2
d y  dy  e x  sinc x  c cosx   e x  cosc x c sin  x
dx 2 dx 1 2 1 2
dy  dy 
    y   y by(1)and(2) 
dx  dx 

2
d y dy
or  2  2y  0
dx 2 dx
which is the required differential equation.

Notes The differential equation of two arbitrary constants family is attained by
differentiating the equation of the family twice and by eliminating the arbitrary constants.

Example: Form a differential equation to represent the family of curves y = A cos x +
B sin x
Solution:
Since, y = A cos x + B sin x

dy
 A sin + B cos x
x
dx
2
d y
 A cos + B sin  (A cos + B sin )
x
x
x
x
dx 2
 y
2
d y
i.e., 2 + y = 0.
dx
Hence, the required differential equation is
2
d y + y = 0.
dx 2

Example: Form a differential equation of the family of curves y = Aex +  Be 2x for different
values of A and B.
Solution:
Given, y = Aex +  Be 2x ...(1)

LOVELY PROFESSIONAL UNIVERSITY 107