Page 26 - DMTH202_BASIC_MATHEMATICS_II

Unit 2: Integration by Partial Fraction

Notes
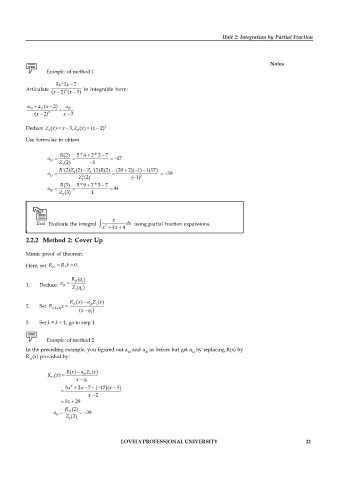
Example: of method 1

5x 2 2x  7
Articulate 2 in integrable form:
(x  2) (x  3)

a  a (x  2) a
10 11  20
(x  2) 2 x  3

x

x
Deduce Z ( )   3,Z ( ) (x  2) 2
x
1 2
Use formulae to obtain
R (2) 5 * 4 2 * 2 7


a     17
10
Z 1 (2)  1
R
Z
R '(2) (2) Z '(2) (2) (20 2)( 1) 1(17)




a  1 1    39
11
Z 2 1 (2) ( 1) 2

R (3) 5 * 9 2 * 3 7


a    44
20
Z (3) 1
2
x
Task Evaluate the integral  dx using partial fraction expansions.
2
x  4x  4
2.2.2 Method 2: Cover Up
Mimic proof of theorem:
Here, set R  , R k  .
0
0 j
R jk ( )
q
j
1. Deduce: a  Z ( )
jk
q
j j
x
x

R ( ) a Z ( )
2. Set R ( j k 1) x  jk jk j

(x q j )
3. Set k = k + 1, go to step 1.
Example: of method 2
In the preceding example, you figured out a and a as before but get a by replacing R(x) by
10 20 11
R (x) provided by:
11
R ( ) a Z ( )
x
x

R 11 ( )  10 1
x
x q 1

2
5x  2x  7 ( 17)(x  3)



x  2
 5x  29
R (2)
a  11   39
11
Z (2)
1
LOVELY PROFESSIONAL UNIVERSITY 21