Page 181 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
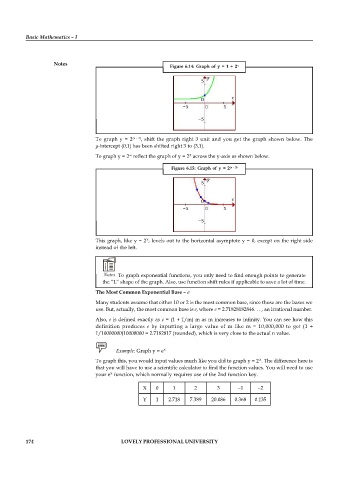
Figure 6.14: Graph of y = 1 + 2 x

To graph y = 2 (x - 3) , shift the graph right 3 unit and you get the graph shown below. The
y-intercept (0,1) has been shifted right 3 to (3,1).
-x
X
To graph y = 2 reflect the graph of y = 2 across the y-axis as shown below.
Figure 6.15: Graph of y = 2 (x - 3)

X
This graph, like y = 2 , levels out to the horizontal asymptote y = 0, except on the right side
instead of the left.

Notes To graph exponential functions, you only need to find enough points to generate
the “L” shape of the graph. Also, use function shift rules if applicable to save a lot of time.

The Most Common Exponential Base – e
Many students assume that either 10 or 2 is the most common base, since those are the bases we
use. But, actually, the most common base is e, where e = 2.71828182846. . . , an irrational number.

Also, e is defined exactly as e = (1 + 1/m) m as m increases to infinity. You can see how this
definition produces e by inputting a large value of m like m = 10,000,000 to get (1 +
1/10000000)10000000 = 2.7182817 (rounded), which is very close to the actual n value.

Example: Graph y = e X

X
To graph this, you would input values much like you did to graph y = 2 . The difference here is
that you will have to use a scientific calculator to find the function values. You will need to use
X
your e function, which normally requires use of the 2nd function key.
X 0 1 2 3 1 2
Y 1 2.718 7.389 20.086 0.368 0.135

174 LOVELY PROFESSIONAL UNIVERSITY