Page 182 - DMTH201_Basic Mathematics-1

Unit 6: Functions

Notes
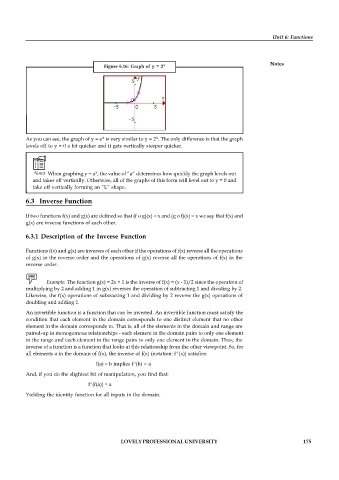
Figure 6.16: Graph of y = 2 X

X
X
As you can see, the graph of y = e is very similar to y = 2 . The only difference is that the graph
levels off to y = 0 a bit quicker and it gets vertically steeper quicker.

Notes When graphing y = a , the value of “a” determines how quickly the graph levels out
X
and takes off vertically. Otherwise, all of the graphs of this form will level out to y = 0 and
take off vertically forming an “L” shape.

6.3 Inverse Function

If two functions f(x) and g(x) are defined so that (f o g)(x) = x and (g o f)(x) = x we say that f(x) and
g(x) are inverse functions of each other.

6.3.1 Description of the Inverse Function

Functions f(x) and g(x) are inverses of each other if the operations of f(x) reverse all the operations
of g(x) in the reverse order and the operations of g(x) reverse all the operations of f(x) in the
reverse order.

Example: The function g(x) = 2x + 1 is the inverse of f(x) = (x - 1)/2 since the operation of
multiplying by 2 and adding 1 in g(x) reverses the operation of subtracting 1 and dividing by 2.
Likewise, the f(x) operations of subtracting 1 and dividing by 2 reverse the g(x) operations of
doubling and adding 1.
An invertible function is a function that can be inverted. An invertible function must satisfy the
condition that each element in the domain corresponds to one distinct element that no other
element in the domain corresponds to. That is, all of the elements in the domain and range are
paired-up in monogomous relationships - each element in the domain pairs to only one element
in the range and each element in the range pairs to only one element in the domain. Thus, the
inverse of a function is a function that looks at this relationship from the other viewpoint. So, for
-1
all elements a in the domain of f(x), the inverse of f(x) (notation: f (x)) satisfies:
-1
f(a) = b implies f (b) = a
And, if you do the slightest bit of manipulation, you find that:
f (f(a)) = a
-1
Yielding the identity function for all inputs in the domain.

LOVELY PROFESSIONAL UNIVERSITY 175