Page 187 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes

Now that we think of f as “acting on” numbers and transforming them, we can define the inverse
of f as the function that “undoes” what f did. In other words, the inverse of f needs to take 7 back
to 3, and take -3 back to -2, etc.

Let g(x) = (x - 1)/2. Then g(7) = 3, g(-3) = -2, and g(11) = 5, so g seems to be undoing what f did, at
least for these three values. To prove that g is the inverse of f we must show that this is true for
any value of x in the domain of f. In other words, g must take f(x) back to x for all values of x in
the domain of f. So, g(f(x)) = x must hold for all x in the domain of f. The way to check this
condition is to see that the formula for g(f(x)) simplifies to x.
g(f(x)) = g(2x + 1) = (2x + 1 -1)/2 = 2x/2 = x.
This simplification shows that if we choose any number and let f act it, then applying g to the
result recovers our original number. We also need to see that this process works in reverse, or
that f also undoes what g does.
f(g(x)) = f((x - 1)/2) = 2(x - 1)/2 + 1 = x - 1 + 1 = x.

-1
-1
Letting f denote the inverse of f, we have just shown that g = f .
6.3.3 Graphs of Inverse Functions

We have seen examples of reflections in the plane. The reflection of a point (a,b) about the x-axis
is (a, -b), and the reflection of (a, b) about the y-axis is (-a, b). Now we want to reflect about the
line y = x.
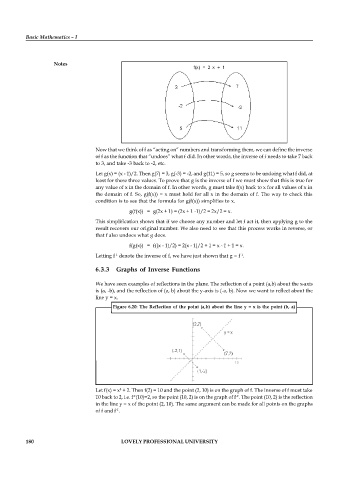
Figure 6.20: The Reflection of the point (a,b) about the line y = x is the point (b, a)

3
Let f(x) = x + 2. Then f(2) = 10 and the point (2, 10) is on the graph of f. The inverse of f must take
-1
-1
10 back to 2, i.e. f (10)=2, so the point (10, 2) is on the graph of f . The point (10, 2) is the reflection
in the line y = x of the point (2, 10). The same argument can be made for all points on the graphs
-1
of f and f .

180 LOVELY PROFESSIONAL UNIVERSITY