Page 192 - DMTH201_Basic Mathematics-1

Unit 6: Functions

Notes
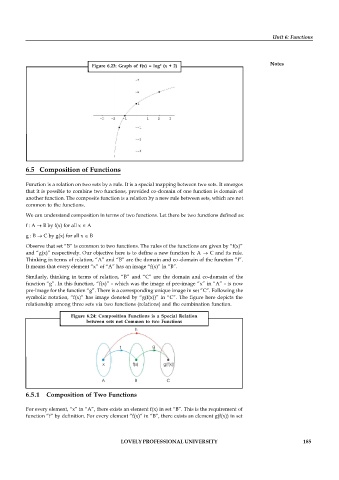
Figure 6.23: Graph of f(x) = log (x + 2)
2

6.5 Composition of Functions

Function is a relation on two sets by a rule. It is a special mapping between two sets. It emerges
that it is possible to combine two functions, provided co-domain of one function is domain of
another function. The composite function is a relation by a new rule between sets, which are not
common to the functions.

We can understand composition in terms of two functions. Let there be two functions defined as:
f : A B by f(x) for all x A
g : B C by g(x) for all x B

Observe that set “B” is common to two functions. The rules of the functions are given by “f(x)”
and “g(x)” respectively. Our objective here is to define a new function h: A C and its rule.
Thinking in terms of relation, “A” and “B” are the domain and co-domain of the function “f”.
It means that every element “x” of “A” has an image “f(x)” in “B”.
Similarly, thinking in terms of relation, “B” and “C” are the domain and co-domain of the
function “g”. In this function, “f(x)” - which was the image of pre-image “x” in “A” - is now
pre-image for the function “g”. There is a corresponding unique image in set “C”. Following the
symbolic notation, “f(x)” has image denoted by “g(f(x))” in “C”. The figure here depicts the
relationship among three sets via two functions (relations) and the combination function.

Figure 6.24: Composition Functions is a Special Relation
between sets not Common to two Functions

6.5.1 Composition of Two Functions

For every element, “x” in “A”, there exists an element f(x) in set “B”. This is the requirement of
function “f” by definition. For every element “f(x)” in “B”, there exists an element g(f(x)) in set

LOVELY PROFESSIONAL UNIVERSITY 185