Page 188 - DMTH201_Basic Mathematics-1
P. 188
Unit 6: Functions
Notes
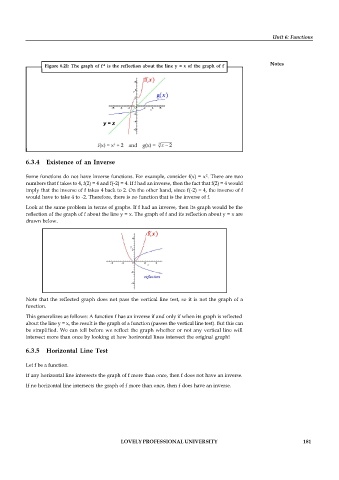
Figure 6.21: The graph of f is the reflection about the line y = x of the graph of f
-1
6.3.4 Existence of an Inverse
Some functions do not have inverse functions. For example, consider f(x) = x . There are two
2
numbers that f takes to 4, f(2) = 4 and f(-2) = 4. If f had an inverse, then the fact that f(2) = 4 would
imply that the inverse of f takes 4 back to 2. On the other hand, since f(-2) = 4, the inverse of f
would have to take 4 to -2. Therefore, there is no function that is the inverse of f.
Look at the same problem in terms of graphs. If f had an inverse, then its graph would be the
reflection of the graph of f about the line y = x. The graph of f and its reflection about y = x are
drawn below.
Note that the reflected graph does not pass the vertical line test, so it is not the graph of a
function.
This generalizes as follows: A function f has an inverse if and only if when its graph is reflected
about the line y = x, the result is the graph of a function (passes the vertical line test). But this can
be simplified. We can tell before we reflect the graph whether or not any vertical line will
intersect more than once by looking at how horizontal lines intersect the original graph!
6.3.5 Horizontal Line Test
Let f be a function.
If any horizontal line intersects the graph of f more than once, then f does not have an inverse.
If no horizontal line intersects the graph of f more than once, then f does have an inverse.
LOVELY PROFESSIONAL UNIVERSITY 181