Page 194 - DMTH201_Basic Mathematics-1

Unit 6: Functions

Solution: Notes
Here,
Domain of “f” = {1,2,3,4}
Range of “f” = {2,3,4,5}

Domain of “g” = {2,3,4,5}
Range of “g” = {4,2,3,1} = {1,2,3,4}
Hence,
Range of “f” Domain of “g” and

Range of “g” Domain of “f”
It means that both compositions “g o f” and “f o g” exist for the given sets.

Another Method of Explanation

Consider the two functions given below:
y = 2x + 1, x {1,2,3}
z = y + 1, y {3,5,7}

Then z is the composition of two functions x and y because z is defined in terms of y and y in terms
of x.

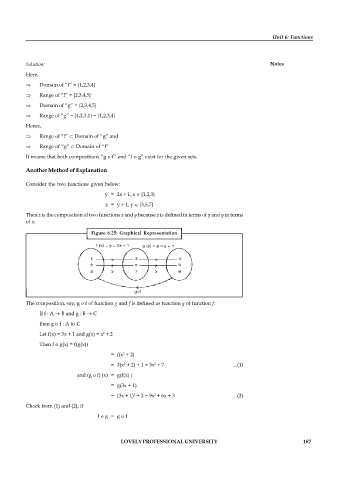
Figure 6.25: Graphical Representation

The composition, say, g o f of function g and f is defined as function g of function f.
If f : A B and g : B C
then g o f : A to C

2
Let f(x) = 3x + 1 and g(x) = x + 2
Then f o g(x) = f(g(x))
= f(x + 2)
2
2
2
= 3(x + 2) + 1 = 3x + 7 …(1)
and (g o f) (x) = g(f(x) )
= g(3x + 1)
2
2
= (3x + 1) + 2 = 9x + 6x + 3 …(2)
Check from (1) and (2), if
f o g = g o f

LOVELY PROFESSIONAL UNIVERSITY 187