Page 297 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
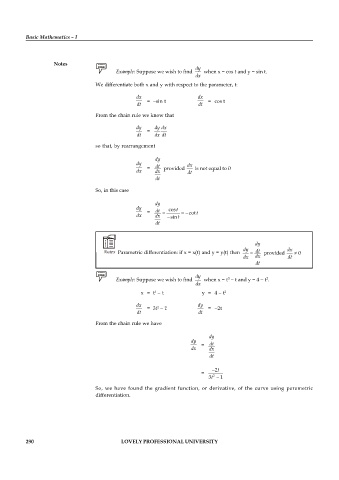
dy
Example: Suppose we wish to find when x = cos t and y = sin t.
dx
We differentiate both x and y with respect to the parameter, t:

dx dx
= sin t = cos t
dt dt
From the chain rule we know that

dy dy dx
=
dt dx dt
so that, by rearrangement
dy
dy dx
= dt provided is not equal to 0
dx dx dt
dt
So, in this case

dy
dy cost
= dt cott
dx dx sint
dt

dy
dy dx
Notes Parametric differentiation: if x = x(t) and y = y(t) then dt provided 0
dx dx dt
dt

dy
2
3
Example: Suppose we wish to find when x = t t and y = 4 t .
dx
3
x = t t y = 4 t 2
dx dy
= 3t 1 = 2t
2
dt dt
From the chain rule we have
dy
dy
= dt
dx dx
dt

2t
=
3t 2 1
So, we have found the gradient function, or derivative, of the curve using parametric
differentiation.

290 LOVELY PROFESSIONAL UNIVERSITY