Page 304 - DMTH201_Basic Mathematics-1
P. 304
Unit 11: Parametric Differentiation
Notes
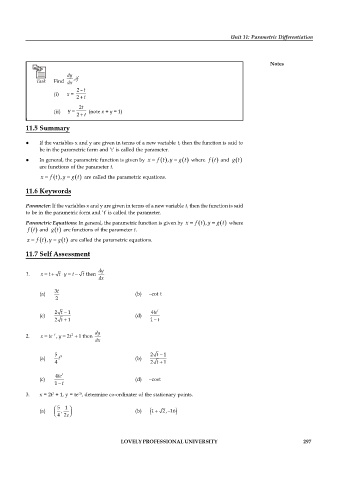
dy
Task Find dx ,if
2 – t
(i) x =
2 t
2t
(ii) y (note x + y = 1)
2 t
11.5 Summary
If the variables x and y are given in terms of a new variable t, then the function is said to
be in the parametric form and ‘t’ is called the parameter.
In general, the parametric function is given by x f t ,y g t where f t and g t
are functions of the parameter t.
x f t ,y g t are called the parametric equations.
11.6 Keywords
Parameter: If the variables x and y are given in terms of a new variable t, then the function is said
to be in the parametric form and ‘t’ is called the parameter.
Parametric Equations: In general, the parametric function is given by x f t ,y g t where
f t and g t are functions of the parameter t.
x f t ,y g t are called the parametric equations.
11.7 Self Assessment
dy
1. x t t y t t then
dx
3t
(a) (b) cot t
2
2 t 1 4te t
(c) (d)
2 t 1 1 t
dy
2. x te t , y 2t 2 1 then
dx
5 3 2 t 1
(a) t (b)
4 2 t 1
4te t
(c) (d) cost
1 t
3
3. x = 2t + 1, y = te , determine co-ordinater of the stationary points.
-2t
5 1
(a) , (b) 1 2, 16
4 2e
LOVELY PROFESSIONAL UNIVERSITY 297