Page 338 - DMTH201_Basic Mathematics-1
P. 338
Unit 13: Maxima and Minima
Notes
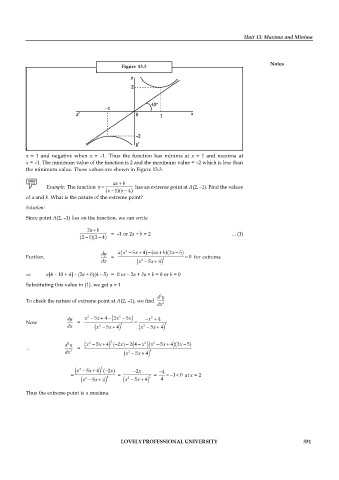
Figure 13.3
x = 1 and negative when x = –1. Thus the function has minima at x = 1 and maxima at
x = –1. The minimum value of the function is 2 and the maximum value = –2 which is less than
the minimum value. These values are shown in Figure 13.3.
ax b
Example: The function y has an extreme point at A(2, –1). Find the values
x 1 x 4
of a and b. What is the nature of the extreme point?
Solution:
Since point A(2, –1) lies on the function, we can write
2a b
= –1 or 2a + b = 2 ... (1)
2 1 2 4
dy a x 2 5x 4 ax b 2x 5
Further, = 0 for extrema
dx x 2 5x 4 2
a[4 – 10 + 4] – (2a + b)(4 – 5) = 0 or – 2a + 2a + b = 0 or b = 0
Substituting this value in (1), we get a = 1
2
d y
To check the nature of extreme point at A(2, –1), we find 2
dx
dy x 2 5x 4 2x 2 5x x 2 4
Now =
dx x 2 5x 4 2 x 2 5x 4 2
2 2 2
2
2
d y x 5x 4 2x 2 4 x x 5x 4 2x 5
=
dx 2 x 2 5x 4 4
x 2 5x 4 2 2x 2x 4
= 4 = 2 2 = 1 0 at x = 2
x 2 5x 4 x 5x 4 4
Thus the extreme point is a maxima.
LOVELY PROFESSIONAL UNIVERSITY 331