Page 341 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
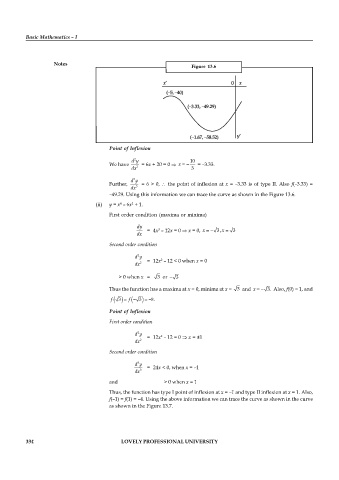
Figure 13.6

Point of Inflexion

2
d y 10
We have = 6x + 20 = 0 x = –3.33.
dx 2 3
3
d y
Further, = 6 > 0, the point of inflexion at x = –3.33 is of type II. Also f(–3.33) =
dx 3
–49.29. Using this information we can trace the curve as shown in the Figure 13.6.
4
(ii) y = x – 6x + 1.
2
First order condition (maxima or minima)
dy
3
= 4x – 12x = 0 x = 0, x 3, x 3
dx
Second order condition

2
d y
2
= 12x – 12 < 0 when x = 0
dx 2
> 0 when x = 3 or 3
Thus the function has a maxima at x = 0, minima at x = 3 and x 3. Also, f(0) = 1, and
f 3 f 3 8.

Point of Inflexion
First order condition

2
d y
2
= 12x – 12 = 0 x = ±1
dx 2
Second order condition
3
d y
= 24x < 0, when x = –1
dx 3
and > 0 when x = 1

Thus, the function has type I point of inflexion at x = –1 and type II inflexion at x = 1. Also,
f(–1) = f(1) = –4. Using the above information we can trace the curve as shown in the curve
as shown in the Figure 13.7.

334 LOVELY PROFESSIONAL UNIVERSITY