Page 38 - DMTH201_Basic Mathematics-1

Unit 2: Trigonometric Functions-II

2.4 Inverse of a Trigonometric Function Notes

In the previous lesson, you have studied the definition of a function and different kinds of
functions. We have defined inverse function.

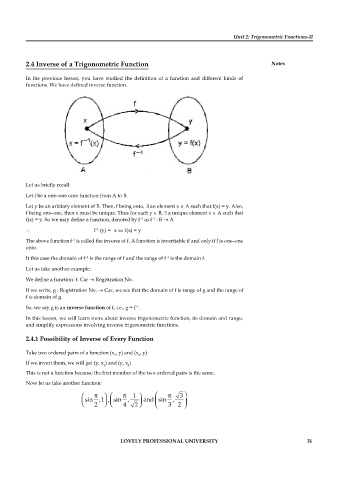
Let us briefly recall:

Let f be a one–one onto function from A to B.

Let y be an arbitary element of B. Then, f being onto, an element x A such that f(x) = y. Also,
f being one–one, then x must be unique. Thus for each y B, a unique element x A such that
–1

f(x) = y. So we may define a function, denoted by f as f : B A
–1
–1
f (y) = x f(x) = y
–1
The above function f is called the inverse of f. A function is invertiable if and only if f is one–one
onto.
–1
–1
It this case the domain of f is the range of f and the range of f is the domain f.
Let us take another example.

We define a function: f: Car Registration No.
If we write, g : Registration No. Car, we see that the domain of f is range of g and the range of
f is domain of g.
–1
So, we say g is an inverse function of f, i.e., g = f .
In this lesson, we will learn more about inverse trigonometric function, its domain and range,
and simplify expressions involving inverse trigonometric functions.

2.4.1 Possibility of Inverse of Every Function

Take two ordered pairs of a function (x , y) and (x , y)
1 2
If we invert them, we will get (y, x ) and (y, x )
1 2
This is not a function because the first member of the two ordered pairs is the same.

Now let us take another function:

LOVELY PROFESSIONAL UNIVERSITY 31