Page 59 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
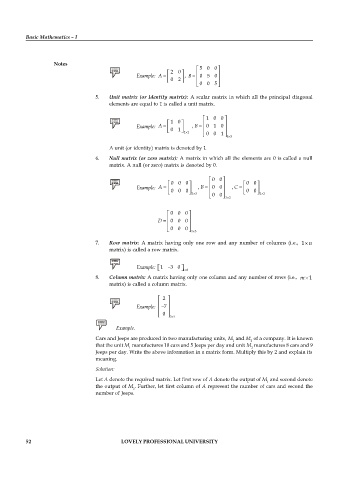
5 0 0
2 0
Example: A , B 0 5 0
0 2
0 0 5
5. Unit matrix (or Identity matrix): A scalar matrix in which all the principal diagonal
elements are equal to 1 is called a unit matrix.

1 0 0
1 0
Example: A , B 0 1 0
0 1
2 2 0 0 1
3 3
A unit (or identity) matrix is denoted by I.
6. Null matrix (or zero matrix): A matrix in which all the elements are 0 is called a null
matrix. A null (or zero) matrix is denoted by 0.

0 0
0 0 0 0 0
Example: A , B 0 0 , C
0 0 0 0 0
2 3 0 0 2 2
3 2
0 0 0
D 0 0 0
0 0 0
3 3
7. Row matrix: A matrix having only one row and any number of columns (i.e., 1 n
matrix) is called a row matrix.

Example: 1 3 0
1 3
8. Column matrix: A matrix having only one column and any number of rows (i.e., m 1
matrix) is called a column matrix.

2
Example: 7
0
3 1

Example.
Cars and Jeeps are produced in two manufacturing units, M and M of a company. It is known
1 2
that the unit M manufactures 10 cars and 5 Jeeps per day and unit M manufactures 8 cars and 9
1 2
Jeeps per day. Write the above information in a matrix form. Multiply this by 2 and explain its
meaning.
Solution:

Let A denote the required matrix. Let first row of A denote the output of M and second denote
1
the output of M . Further, let first column of A represent the number of cars and second the
2
number of Jeeps.

52 LOVELY PROFESSIONAL UNIVERSITY