Page 111 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

Notes
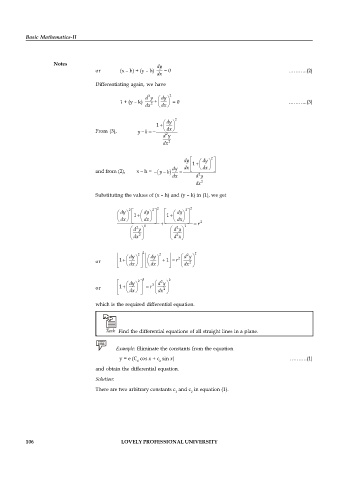
dy
or (x – h) + (y – h) = 0 ………..(2)
dx
Differentiating again, we have

2
d y  dy  2
1 + (y – k) 2    = 0 ………..(3)
dx  dx 
2
 dy 
1   
From (3), y     dx 
k
2
d y
dx 2
dy   dy  2 
1    
dy dx    dx   
and from (2), x – h =   y k  

2
dx d y
dx 2
Substituting the values of (x – h) and (y – k) in (1), we get

2 2 2 2 2
 dy    dy     dy  
  1     1    
 dx     dx      dx    2
 1 r
1
2
2
 d y   d y 
 2   2 
 dx   d x 
2 2
2
  dy  2   dy  2  2  d y 

or 1         1  r  2 
   dx      dx     dx 

2
  dy  2  3 2  d y  2
or 1      r  2 
   dx     dx 
which is the required differential equation.

Task Find the differential equations of all straight lines in a plane.

Example: Eliminate the constants from the equation
y = e (C cos x + c sin x) ……….(1)
1 2
and obtain the differential equation.

Solution:
There are two arbitrary constants c and c in equation (1).
1 2

106 LOVELY PROFESSIONAL UNIVERSITY