Page 114 - DMTH202_BASIC_MATHEMATICS_II

Unit 8: Formation of Differential Equation

Notes
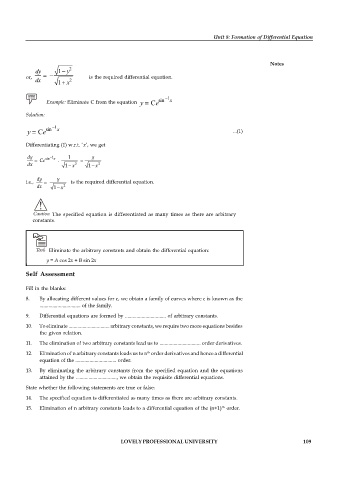
2
dy 1 y
or,   is the required differential equation.
dx 1 x 2

1

Example: Eliminate C from the equation y  Ce sin x
Solution:

 1
sin x
y  Ce ...(1)
Differentiating (1) w.r.t. ‘x’, we get

dy sin  1 x 1 y
 Ce . 
dx 1 x 2 1 x 2


dy y
i.e.,  is the required differential equation.
dx 1 x 2

!
Caution The specified equation is differentiated as many times as there are arbitrary
constants.

Task Eliminate the arbitrary constants and obtain the differential equation:

y = A cos 2x + B sin 2x

Self Assessment

Fill in the blanks:
8. By allocating different values for c, we obtain a family of curves where c is known as the
................................ of the family.

9. Differential equations are formed by ................................ of arbitrary constants.
10. To eliminate ................................ arbitrary constants, we require two more equations besides
the given relation.

11. The elimination of two arbitrary constants lead us to ................................ order derivatives.
12. Elimination of n arbitrary constants leads us to n order derivatives and hence a differential
th
equation of the ................................ order.
13. By eliminating the arbitrary constants from the specified equation and the equations
attained by the ................................, we obtain the requisite differential equations.
State whether the following statements are true or false:

14. The specified equation is differentiated as many times as there are arbitrary constants.
15. Elimination of n arbitrary constants leads to a differential equation of the (n+1) order.
th

LOVELY PROFESSIONAL UNIVERSITY 109