Page 194 - DMTH202_BASIC_MATHEMATICS_II

Unit 14: Probability

Notes
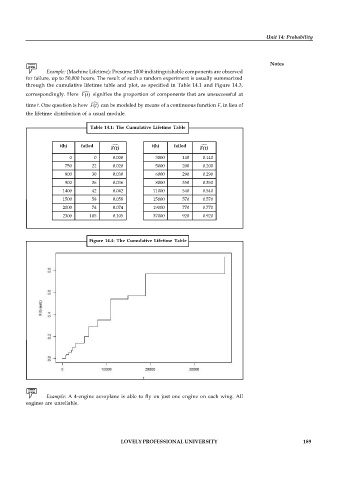
Example: (Machine Lifetime): Presume 1000 indistinguishable components are observed
for failure, up to 50,000 hours. The result of such a random experiment is usually summarized
through the cumulative lifetime table and plot, as specified in Table 14.1 and Figure 14.3,

correspondingly. Here ( )F t signifies the proportion of components that are unsuccessful at

time t. One question is how ( )F t can be modeled by means of a continuous function F, in lieu of
the lifetime distribution of a usual module.

Table 14.1: The Cumulative Lifetime Table

t(h) failed  t(h) failed 
F(t) F(t)
0 0 0.000 3000 140 0.140
750 22 0.020 5000 200 0.200
800 30 0.030 6000 290 0.290
900 36 0.036 8000 350 0.350
1400 42 0.042 11000 540 0.540
1500 58 0.058 15000 570 0.570
2000 74 0.074 19000 770 0.770
2300 105 0.105 37000 920 0.920

Figure 14.4: The Cumulative Lifetime Table

Example: A 4-engine aeroplane is able to fly on just one engine on each wing. All
engines are unreliable.

LOVELY PROFESSIONAL UNIVERSITY 189