Page 198 - DMTH202_BASIC_MATHEMATICS_II

Unit 14: Probability

In this Venn diagram we observe Notes
(i) A  C =  and consequently events A and C are disjoint.
(ii) (A Bc)  (Ac  B) =  and therefore events A  Bc and Ac B are disjoint.

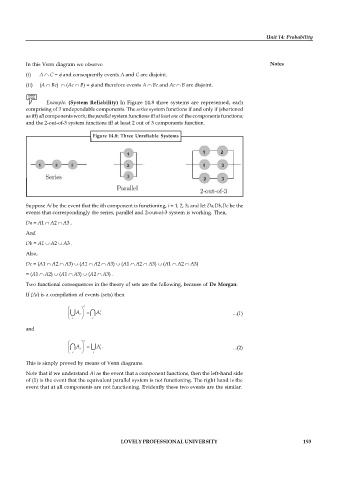
Example: (System Reliability) In Figure 14.8 three systems are represented, each
comprising of 3 undependable components. The series system functions if and only if (shortened
as iff) all components work; the parallel system functions iff at least one of the components functions;
and the 2-out-of-3 system functions iff at least 2 out of 3 components function.

Figure 14.8: Three Unreliable Systems

Suppose Ai be the event that the ith component is functioning, i = 1, 2, 3; and let Da,Db,Dc be the
events that correspondingly the series, parallel and 2-out-of-3 system is working. Then,
Da = A1  A2  A3 ,

And
Db = A1  A2  A3 .
Also,
Dc = (A1  A2  A3)  (A1  A2  A3)  (A1  A2  A3)  (A1  A2  A3)

= (A1  A2)  (A1  A3)  (A2  A3) .
Two functional consequences in the theory of sets are the following, because of De Morgan:
If {Ai} is a compilation of events (sets) then

c
 
 A i   A i c ...(1)

 i  i
and
c
 
 A i   A c i . ...(2)

 i  i
This is simply proved by means of Venn diagrams.
Note that if we understand Ai as the event that a component functions, then the left-hand side
of (1) is the event that the equivalent parallel system is not functioning. The right hand is the
event that at all components are not functioning. Evidently these two events are the similar.

LOVELY PROFESSIONAL UNIVERSITY 193