Page 43 - DMGT409Basic Financial Management

Basic Financial Management

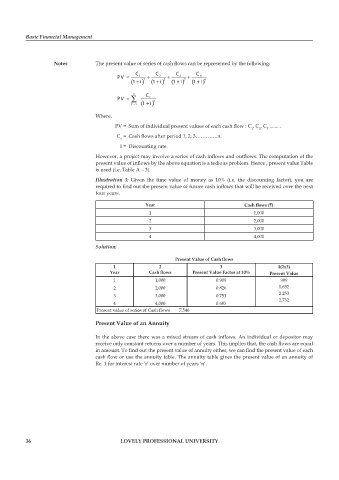
Notes The present value of series of cash flows can be represented by the following:

C C C C
PV = 1 + 2 + 3 + n
1
1
1
( + i ) 1 ( + i ) 2 ( + i ) 3 ( + i ) n
1
n C
PV = ∑ t n
1
T =1 ( + i )
Where,
PV = Sum of individual present values of each cash flow : C , C , C ..........

1 2 3
C = Cash fl ows after period 1, 2, 3………….n.
n
I = Discounting rate.

However, a project may involve a series of cash inflows and outflows. The computation of the

present value of inflows by the above equation is a tedious problem. Hence , present value Table

is used (i.e. Table A – 3).
Illustration 3: Given the time value of money as 10% (i.e. the discounting factor), you are

required to find out the present value of future cash inflows that will be received over the next

four years.
Year Cash fl ows (`)
1 1,000
2 2,000
3 3,000
4 4,000
Solution:

Present Value of Cash fl ows
1 2 3 4(2x3)
Year Cash fl ows Present Value Factor at 10% Present Value
1 1,000 0.909 909
2 2,000 0.826 1,652
2.253
3 3,000 0.751
2,732
4 4,000 0.683
Present value of series of Cash flows 7,546

Present Value of an Annuity

In the above case there was a mixed stream of cash inflows. An individual or depositor may
receive only constant returns over a number of years. This implies that, the cash flows are equal

in amount. To find out the present value of annuity either, we can find the present value of each

cash flow or use the annuity table. The annuity table gives the present value of an annuity of

Re. 1 for interest rate ‘r’ over number of years ‘n’.

36 LOVELY PROFESSIONAL UNIVERSITY