Page 44 - DMGT409Basic Financial Management

Unit 3: Time Value of Money

Notes
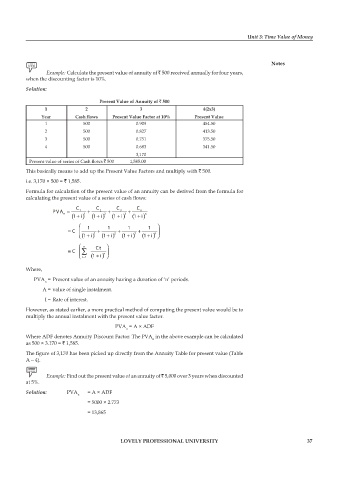
Example: Calculate the present value of annuity of ` 500 received annually for four years,
when the discounting factor is 10%.
Solution:

Present Value of Annuity of ` 500
1 2 3 4(2x3)
Year Cash fl ows Present Value Factor at 10% Present Value
1 500 0.909 454.50
2 500 0.827 413.50
3 500 0.751 375.50
4 500 0.683 341.50
3,170
Present value of series of Cash fl ows ` 500 1,585.00
This basically means to add up the Present Value Factors and multiply with ` 500.
i.e. 3,170 × 500 = ` 1,585.
Formula for calculation of the present value of an annuity can be derived from the formula for
calculating the present value of a series of cash fl ows:
C C C C
PVA n = 1 + 2 + 3 + n
1
1
1
( + i ) 1 ( + i ) 2 ( + i ) 3 ( + i ) n
1
⎛ 1 1 1 1 ⎞
= C ⎜ 1 + 2 + 3 + n ⎟
1
1
1
( + i
⎝ 1 ) ( + i ) ( + i ) ( + i ) ⎠
⎛ n Ct ⎞
= C ⎜∑ n ⎟
1
⎝ t =1 ( + i ) ⎠
Where,
PVA = Present value of an annuity having a duration of ‘n’ periods.
n
A = value of single instalment.
I = Rate of interest.
However, as stated earlier, a more practical method of computing the present value would be to
multiply the annual instalment with the present value factor.
PVA = A × ADF
n
Where ADF denotes Annuity Discount Factor. The PVA in the above example can be calculated
n
as 500 × 3.170 = ` 1,585.

The figure of 3,170 has been picked up directly from the Annuity Table for present value (Table
A – 4).

Example: Find out the present value of an annuity of ` 5,000 over 3 years when discounted
at 5%.
Solution: PVA = A × ADF
n
= 5000 × 2.773
= 13,865

LOVELY PROFESSIONAL UNIVERSITY 37