Page 51 - DMGT409Basic Financial Management

Basic Financial Management

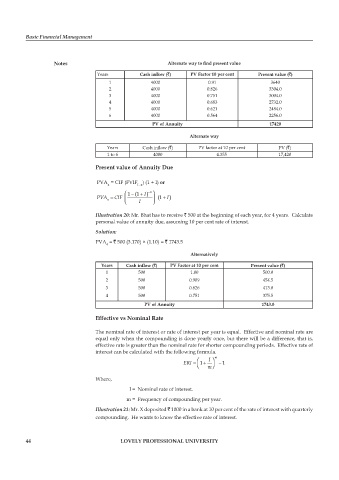
Notes Alternate way to find present value

Years Cash infl ow (`) PV Factor 10 per cent Present value (`)
1 4000 0.91 3640
2 4000 0.826 3304.0
3 4000 0.751 3004.0
4 4000 0.683 2732.0
5 4000 0.621 2484.0
6 4000 0.564 2256.0
PV of Annuity 17420
Alternate way

Years Cash infl ow (`) PV factor at 10 per cent PV (`)
1 to 6 4000 4.355 17,420

Present value of Annuity Due

PVA = CIF (FVIF ) (1 + I) or
n I . n
−
⎛ 1 − (1 I+ ) ⎞
n
PVA = CIF ⎜ ⎟ ( + ) I
1
n
⎝ I ⎠
Illustration 20: Mr. Bhat has to receive ` 500 at the beginning of each year, for 4 years. Calculate
personal value of annuity due, assuming 10 per cent rate of interest.
Solution:
PVA = ` 500 (3.170) × (1.10) = ` 1743.5
4
Alternatively

Years Cash infl ow (`) PV Factor at 10 per cent Present value (`)
1 500 1.00 500.0
2 500 0.909 454.5
3 500 0.826 413.0
4 500 0.751 375.5
PV of Annuity 1743.0

Effective vs Nominal Rate

The nominal rate of interest or rate of interest per year is equal. Effective and nominal rate are
equal only when the compounding is done yearly once, but there will be a difference, that is,
effective rate is greater than the nominal rate for shorter compounding periods. Effective rate of
interest can be calculated with the following formula.
⎛ I ⎞ m
ERI = ⎜ 1 + ⎟ − 1
⎝ m ⎠
Where,

I = Nominal rate of interest.
m = Frequency of compounding per year.
Illustration 21: Mr. X deposited ` 1000 in a bank at 10 per cent of the rate of interest with quarterly
compounding. He wants to know the effective rate of interest.

44 LOVELY PROFESSIONAL UNIVERSITY