Page 165 - DMTH201_Basic Mathematics-1
P. 165
Basic Mathematics – I
Notes implies that y as an explicit function of x is exactly of the same form as x as an explicit
function of y.
Notes:
(i) Two points with coordinates (a, b) and (b, a) are said to be reflections of one another
(or symmetrical) about the line y = x.
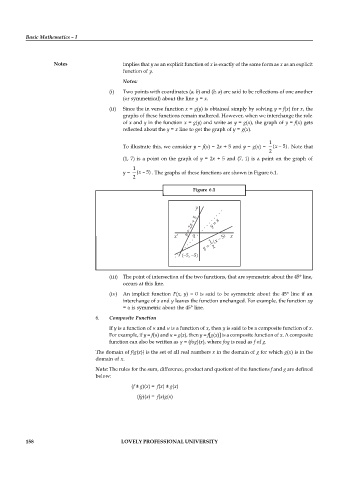
(ii) Since the in verse function x = g(y) is obtained simply by solving y = f(x) for x, the
graphs of these functions remain maltered. However, when we interchange the role
of x and y in the function x = g(y) and write as y = g(x), the graph of y = f(x) gets
reflected about the y = x line to get the graph of y = g(x).
1
x
To illustrate this, we consider y = f(x) = 2x + 5 and y = g(x) = ( – 5) . Note that
2
(1, 7) is a point on the graph of y = 2x + 5 and (7, 1) is a point on the graph of
1
x
y = ( – 5) . The graphs of these functions are shown in Figure 6.1.
2
Figure 6.1
5
2
0 5
1
2
(–5, –5)
(iii) The point of intersection of the two functions, that are symmetric about the 45° line,
occurs at this line.
(iv) An implicit function F(x, y) = 0 is said to be symmetric about the 45° line if an
interchange of x and y leaves the function unchanged. For example, the function xy
= a is symmetric about the 45° line.
6. Composite Function
If y is a function of u and u is a function of x, then y is said to be a composite function of x.
For example, if y = f(u) and u = g(x), then y = f[g(x)] is a composite function of x. A composite
function can also be written as y = (fog)(x), where fog is read as f of g.
The domain of f{g(x)} is the set of all real numbers x in the domain of g for which g(x) is in the
domain of x.
Note: The rules for the sum, difference, product and quotient of the functions f and g are defined
below:
(f ± g)(x) = f(x) ± g(x)
(fg)(x) = f(x)g(x)
158 LOVELY PROFESSIONAL UNIVERSITY