Page 171 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes ax + bx + c = 0
2
x + (b/a)x + c/a = 0
2
Try to get (x+g) = x + (b/a)x + ??
2
2
[x + (b/a)x + b /4a ] b /4a + c/a = 0
2
2
2
2
2
2
[x + (b/2a)] ( b /4a c/a) = 0
2
2
2
2
(x+(1/2)(b/a)) = x + 2(1/2)(b/a)x +
2
2
2
[x + (b/2a)] = b /4a c/a
2
2
(1/4)(b /a )
2
2
2
x+(b/2a) = ±sqrt(b /4a 4ac/4a )
x = b/2a ±sqrt(b 4ac)/2a
2
2
(x+b/2a) = x + (b/a)
2
2
x+(b/2a) = ±sqrt(b /4a 4ac/4a )
2
2
2
x = (-b ±sqrt(b 4ac))/2a

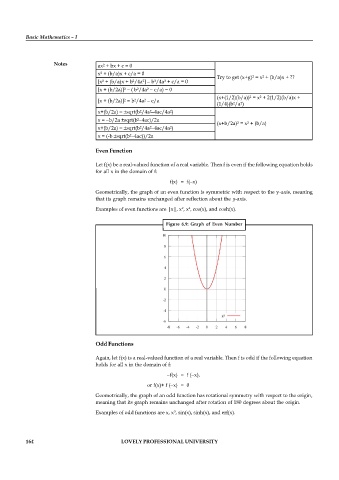
Even Function
Let f(x) be a real-valued function of a real variable. Then f is even if the following equation holds
for all x in the domain of f:
f(x) = f(–x)
Geometrically, the graph of an even function is symmetric with respect to the y-axis, meaning
that its graph remains unchanged after reflection about the y-axis.
4
Examples of even functions are |x|, x , x , cos(x), and cosh(x).
2
Figure 6.9: Graph of Even Number

Odd Functions

Again, let f(x) is a real-valued function of a real variable. Then f is odd if the following equation
holds for all x in the domain of f:
f(x) = f ( x),
or f(x)+ f ( x) = 0
Geometrically, the graph of an odd function has rotational symmetry with respect to the origin,
meaning that its graph remains unchanged after rotation of 180 degrees about the origin.
Examples of odd functions are x, x , sin(x), sinh(x), and erf(x).
3

164 LOVELY PROFESSIONAL UNIVERSITY