Page 18 - DMTH201_Basic Mathematics-1
P. 18
Unit 1: Trigonometric Functions-I
= arccot(x) is the mirror image of the restricted cotangent graph with respect to the line y = x. The Notes
domain is R and the range is [0, ].
Transformations
As with the trigonometric functions, the related functions can be created using simple
transformations.
y = 2.arcsin(x – 1) comes about by moving the graph of arcsin(x) one unit to the right, and then by
multiplying all the images by two. The domain is [0, 2] and the range is [– , ].
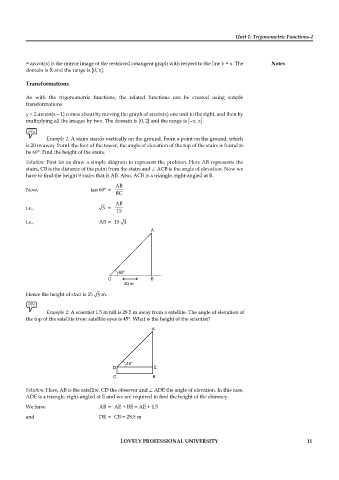
Example 1: A stairs stands vertically on the ground. From a point on the ground, which
is 20 m away from1 the foot of the tower, the angle of elevation of the top of the stairs is found to
be 60°. Find the height of the stairs.
Solution: First let us draw a simple diagram to represent the problem. Here AB represents the
stairs, CB is the distance of the point from the stairs and ACB is the angle of elevation. Now we
have to find the height 9 stairs that is AB. Also, ACB is a triangle, right-angled at B.
Now, tan 60° =
i.e., =
i.e., AB = 15
Hence the height of stair is 15 m.
Example 2: A scientist 1.5 m tall is 28.5 m away from a satellite. The angle of elevation of
the top of the satellite from satellite eyes is 45°. What is the height of the scientist?
Solution: Here, AB is the satellite, CD the observer and ADE the angle of elevation. In this case,
ADE is a triangle, right-angled at E and we are required to find the height of the chimney.
We have AB = AE + BE = AE + 1.5
and DE = CB = 28.5 m
LOVELY PROFESSIONAL UNIVERSITY 11