Page 183 - DMTH201_Basic Mathematics-1
P. 183
Basic Mathematics – I
Notes
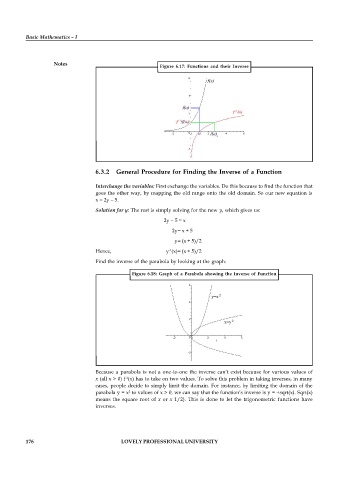
Figure 6.17: Functions and their Inverse
6.3.2 General Procedure for Finding the Inverse of a Function
Interchange the variables: First exchange the variables. Do this because to find the function that
goes the other way, by mapping the old range onto the old domain. So our new equation is
x = 2y 5.
Solution for y: The rest is simply solving for the new y, which gives us:
2y 5 = x
2y= x + 5
y= (x + 5)/2
-1
Hence, y (x)= (x + 5)/2
Find the inverse of the parabola by looking at the graph:
Figure 6.18: Graph of a Parabola showing the Inverse of Function
Because a parabola is not a one-to-one the inverse can’t exist because for various values of
-1
x (all x > 0) f (x) has to take on two values. To solve this problem in taking inverses, in many
cases, people decide to simply limit the domain. For instance, by limiting the domain of the
2
parabola y = x to values of x > 0, we can say that the function’s inverse is y = +sqrt(x). Sqrt(x)
means the square root of x or x 1/2). This is done to let the trigonometric functions have
inverses.
176 LOVELY PROFESSIONAL UNIVERSITY