Page 184 - DMTH201_Basic Mathematics-1

Unit 6: Functions

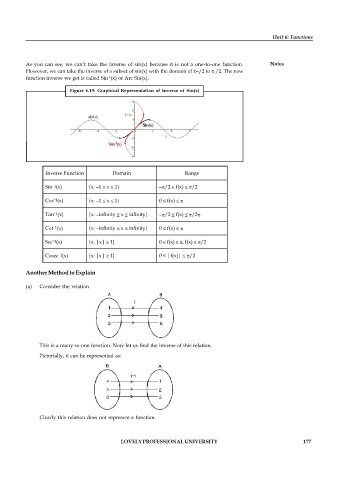
As you can see, we can’t take the inverse of sin(x) because it is not a one-to-one function. Notes
However, we can take the inverse of a subset of sin(x) with the domain of /2 to /2. The new
-1
function inverse we get is called Sin (x) or Arc Sin(x).
Figure 6.19: Graphical Representation of Inverse of Sin(x)

Inverse Function Domain Range
Sin 1 (x) {x: 1 x 1} /2 f(x) /2

Cos 1 (x) {x: 1 x 1} 0 f(x)
Tan 1 (x) {x: infinity x infinity} /2 f(x) /2

Cot 1 (x) {x: infinity x infinity} 0 f(x)

Sec 1 (x) {x: |x| 1} 0 f(x) , f(x) /2

Cosec 1 (x) {x: |x| 1} 0 < |f(x)| /2

Another Method to Explain

(a) Consider the relation

This is a many-to-one function. Now let us find the inverse of this relation.

Pictorially, it can be represented as:

Clearly this relation does not represent a function.

LOVELY PROFESSIONAL UNIVERSITY 177