Page 219 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes Thus we have

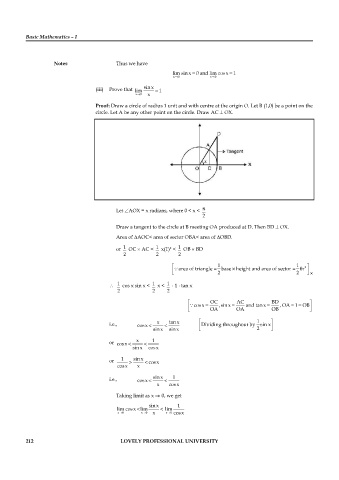
(iii) Prove that

Proof: Draw a circle of radius 1 unit and with centre at the origin O. Let B (1,0) be a point on the
circle. Let A be any other point on the circle. Draw AC OX.

Let AOX = x radians, where 0 < x <

Draw a tangent to the circle at B meeting OA produced at D. Then BD OX.

Area of AOC< area of sector OBA< area of OBD.
2
or OC AC < x(1) < OB BD

×
cos x sin x < x < ∙ 1 ∙ tan x

i.e.,

or

or

i.e.,

Taking limit as x 0, we get

212 LOVELY PROFESSIONAL UNIVERSITY