Page 344 - DMTH201_Basic Mathematics-1

Unit 13: Maxima and Minima

Notes
2
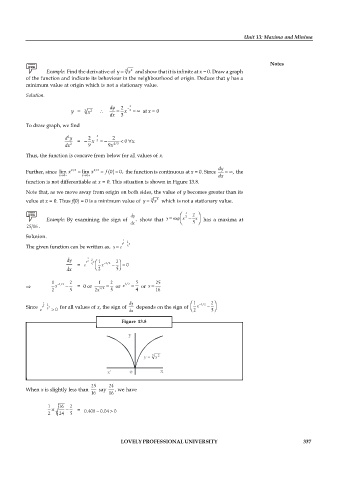
Example: Find the derivative of y 3 x and show that it is infinite at x = 0. Draw a graph
of the function and indicate its behaviour in the neighbourhood of origin. Deduce that y has a
minimum value at origin which is not a stationary value.
Solution.

dy 2 1
y = 3 x 2 x 3 at x = 0
dx 3
To draw graph, we find

2
d y 2 4 2
= x 3 0 x .
dx 2 9 9x 4/3
Thus, the function is concave from below for all values of x.
dy
Further, since lim x 2/3 lim x 2/3 f 0 0, the function is continuous at x = 0. Since , the
x 0 x 0 dx
function is not differentiable at x = 0. This situation is shown in Figure 13.8.

Note that, as we move away from origin on both sides, the value of y becomes greater than its
2
value at x = 0. Thus f(0) = 0 is a minimum value of y 3 x which is not a stationary value.

dy 1 2 2
Example: By examining the sign of dx , show that y exp x 5 x has a maxima at
25 16 .
Solution.
1
x 2 2 x
The given function can be written as, y e 5
dy x 1 2 2 5 x 1 1/2 2
dx = e 2 x 5 0

1 x 1/2 2 1 2 or x 1/2 5 or x 25
2 5 = 0 or 2x 1/2 5 4 16

1 dy 1 1/2 2
Since x 2 2 5 x for all values of x, the sign of depends on the sign of x
e 0 dx 2 5
Figure 13.8

Fig. 5.8
25 24
When x is slightly less than say , we have
16 16
1 16 2
= 0.408 0.04 0
2 24 5

LOVELY PROFESSIONAL UNIVERSITY 337