Page 339 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes 13.2 Points of Inflexion

A point of inflexion marks the change of curvature of a function. Since the curvature may change
from convex (from below) to concave (from below) or vice versa, we have two types of points of
inflexion which would be termed (for convenience) as type I and type II points of inflexion, as
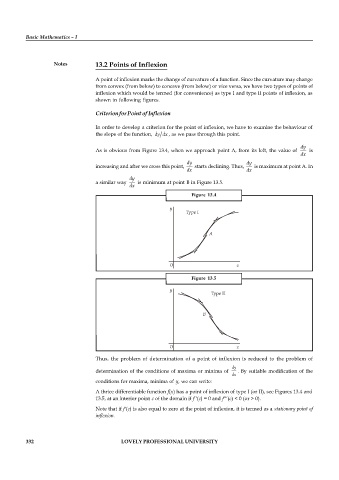
shown in following figures.
Criterion for Point of Inflexion

In order to develop a criterion for the point of inflexion, we have to examine the behaviour of
the slope of the function, dy dx , as we pass through this point.

dy
As is obvious from Figure 13.4, when we approach point A, from its left, the value of is
dx
dy dy
increasing and after we cross this point, starts declining. Thus, is maximum at point A. In
dx dx
dy
a similar way is minimum at point B in Figure 13.5.
dx
Figure 13.4

Figure 13.5

Thus, the problem of determination of a point of inflexion is reduced to the problem of
dy
determination of the conditions of maxima or minima of . By suitable modification of the
dx
conditions for maxima, minima of y, we can write:
A thrice differentiable function f(x) has a point of inflexion of type I (or II), see Figures 13.4 and
13.5, at an interior point c of the domain if f (c) = 0 and f (c) < 0 (or > 0).
Note that if f (c) is also equal to zero at the point of inflexion, it is termed as a stationary point of
inflexion.

332 LOVELY PROFESSIONAL UNIVERSITY