Page 334 - DMTH201_Basic Mathematics-1
P. 334
Unit 13: Maxima and Minima
Notes
Notes 1. The points of the domain at which a function can assume extreme values are
either critical point or end points.
2. The end point(s) can also be a local extrema.
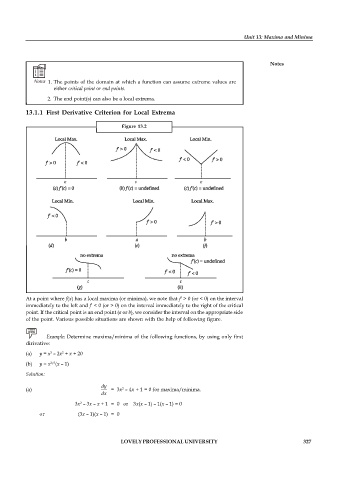
13.1.1 First Derivative Criterion for Local Extrema
Figure 13.2
At a point where f(x) has a local maxima (or minima), we note that f > 0 (or < 0) on the interval
immediately to the left and f < 0 (or > 0) on the interval immediately to the right of the critical
point. If the critical point is an end point (a or b), we consider the interval on the appropriate side
of the point. Various possible situations are shown with the help of following figure.
Example: Determine maxima/minima of the following functions, by using only first
dirivative:
3
2
(a) y = x – 2x + x + 20
(b) y = x (x – 1)
2/3
Solution:
dy
(a) = 3x – 4x + 1 = 0 for maxima/minima.
2
dx
2
3x – 3x – x + 1 = 0 or 3x(x – 1) – 1(x – 1) = 0
or (3x – 1)(x – 1) = 0
LOVELY PROFESSIONAL UNIVERSITY 327