Page 329 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

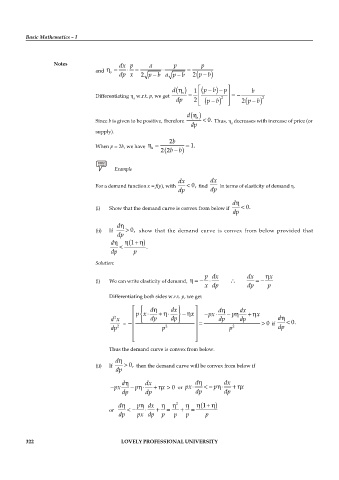
Notes dx p a p p
and s
dp x 2 p b a p b 2 p b

d s 1 p b p b
Differentiating w.r.t. p, we get 2 2
s dp 2 p b 2 p b

d s
Since b is given to be positive, therefore 0. Thus, decreases with increase of price (or
s
dp
supply).
2b
When p = 2b, we have s 1.
2 2b b

Example
dx dx
For a demand function x = f(p), with 0, find in terms of elasticity of demand .
dp dp

d
(i) Show that the demand curve is convex from below if 0.
dp

d
(ii) If 0, show that the demand curve is convex from below provided that
dp
d 1
.
dp p
Solution:

p dx dx x
(i) We can write elasticity of demand,
x dp dp p
Differentiating both sides w.r.t. p, we get

d dx d dx
p x x px p x
2
d x dp dp dp dp d
= 0 if 0.
dp 2 p 2 p 2 dp

Thus the demand curve is convex from below.
d
(ii) If 0, then the demand curve will be convex from below if
dp
d dx d dx
px p x 0 or px p x
dp dp dp dp

d p dx 2 1
or
dp px dp p p p p

322 LOVELY PROFESSIONAL UNIVERSITY