Page 81 - DMTH201_Basic Mathematics-1
P. 81
Basic Mathematics – I
Notes
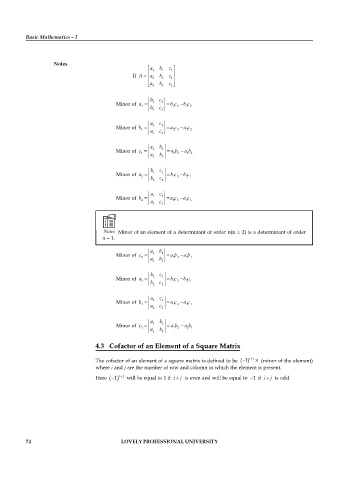
a b c
1 1 1
If A a 2 b 2 c 2
a b c
3 3 3
b 2 c 2
Minor of a b c b c
1 2 3 3 2
b 3 c 3
a c
Minor of b 1 2 2 a c a c
3 2
2 3
a 3 c 3
a b
Minor of c 1 2 2 a b a b
2 3
3 2
a 3 b 3
b 1 c 1
Minor of a 2 b c b c
3 1
1 3
b c
3 3
a 1 c 1
Minor of b 2 a c a c
1 3
3 1
a c
3 3
Notes Minor of an element of a determinant of order n(n 2) is a determinant of order
n 1.
a b
Minor of c 1 1 a b a b
2 1 3 3 1
a 3 b 3
b c
Minor of a 3 1 1 b c b c
1 2
2 1
b 2 c 2
a 1 c 1
Minor of b 3 a c a c
2 1
1 2
a c
2 2
a 1 b 1
Minor of c 3 a b a b
1 2
2 1
a b
2 2
4.3 Cofactor of an Element of a Square Matrix
The cofactor of an element of a square matrix is defined to be ( 1) i j (minor of the element)
where i and j are the number of row and column in which the element is present.
Here ( ) 1 i j will be equal to 1 if i j is even and will be equal to 1 if i j is odd.
74 LOVELY PROFESSIONAL UNIVERSITY