Page 9 - DMTH201_Basic Mathematics-1
P. 9
Basic Mathematics – I
Notes Any time you want to figure out anything to do with angles, or turning, or swinging, there’s
trigonometry involved.
1.1 Trigonometric Coordinates
As you have already studied the trigonometric ratios of acute angles as the ratio of the sides of
a right angled triangle. You have also studied the trigonometric identities and application of
trigonometric ratios in solving the problems related to heights and distances.
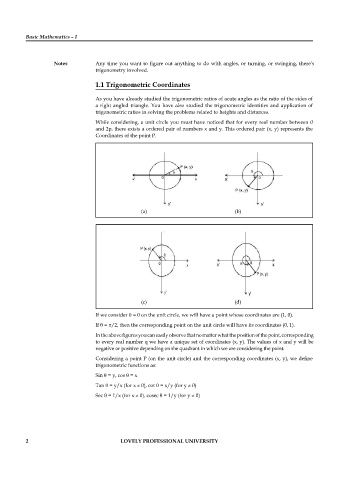
While considering, a unit circle you must have noticed that for every real number between 0
and 2p, there exists a ordered pair of numbers x and y. This ordered pair (x, y) represents the
Coordinates of the point P.
(a) (b)
(c) (d)
If we consider = 0 on the unit circle, we will have a point whose coordinates are (1, 0).
If = /2, then the corresponding point on the unit circle will have its coordinates (0, 1).
In the above figures you can easily observe that no matter what the position of the point, corresponding
to every real number q we have a unique set of coordinates (x, y). The values of x and y will be
negative or positive depending on the quadrant in which we are considering the point.
Considering a point P (on the unit circle) and the corresponding coordinates (x, y), we define
trigonometric functions as:
Sin = y, cos = x
Tan = y/x (for x 0), cot = x/y (for y 0)
Sec = 1/x (for x 0), cosec = 1/y (for y 0)
2 LOVELY PROFESSIONAL UNIVERSITY