Page 143 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 143
Quantitative Techniques – I
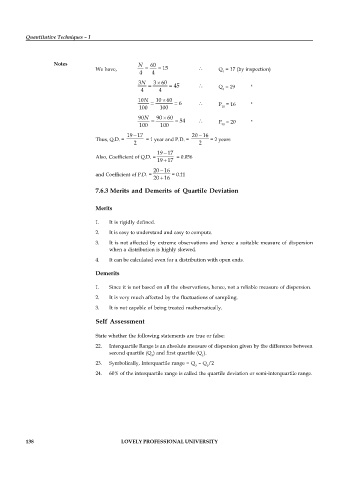
Notes N 60
We have, 15 Q = 17 (by inspection)
4 4 1
3N 3 60
45 Q = 19 "
4 4 3
10N 10 60
6 P = 16 "
100 100 10
90N 90 60
54 P = 20 "
100 100 90
19 17 20 16
Thus, Q.D. = = 1 year and P.D. = = 2 years
2 2
19 17
Also, Coefficient of Q.D. = = 0.056
19 17
20 16
and Coefficient of P.D. = = 0.11
20 16
7.6.3 Merits and Demerits of Quartile Deviation
Merits
1. It is rigidly defined.
2. It is easy to understand and easy to compute.
3. It is not affected by extreme observations and hence a suitable measure of dispersion
when a distribution is highly skewed.
4. It can be calculated even for a distribution with open ends.
Demerits
1. Since it is not based on all the observations, hence, not a reliable measure of dispersion.
2. It is very much affected by the fluctuations of sampling.
3. It is not capable of being treated mathematically.
Self Assessment
State whether the following statements are true or false:
22. Interquartile Range is an absolute measure of dispersion given by the difference between
second quartile (Q ) and first quartile (Q ).
3 1
23. Symbolically, Interquartile range = Q – Q /2
3 1
24. 60% of the interquartile range is called the quartile deviation or semi-interquartile range.
138 LOVELY PROFESSIONAL UNIVERSITY