Page 215 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes Solution:
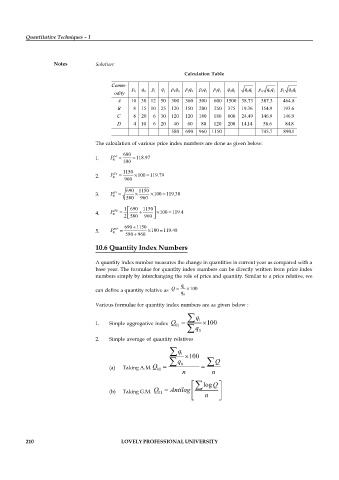
Calculation Table
Comm -
p q p q p q p q p q p q q q q q p q q p q q
odity 0 0 1 1 0 0 1 0 0 1 1 1 0 1 0 1 0 0 1 1 0 1
A 10 30 12 50 300 360 500 600 1500 38.73 387.3 464.8
B 8 15 10 25 120 150 200 250 375 19.36 154.9 193.6
C 6 20 6 30 120 120 180 180 600 24.49 146.9 146.9
D 4 10 6 20 40 60 80 120 200 14.14 56.6 84.8
580 690 960 1150 745.7 890.1

The calculation of various price index numbers are done as given below:
690
1. P 01 La 118.97
580
1150
Pa
2. P 01 100 119.79
960
690 1150
Fi
3. P 01 100 119.38
580 960
1 690 1150
DB
4. P 01 100 119.4
2 580 960
690 1150
ME
5. P 01 100 119.48
580 960
10.6 Quantity Index Numbers

A quantity index number measures the change in quantities in current year as compared with a
base year. The formulae for quantity index numbers can be directly written from price index
numbers simply by interchanging the role of price and quantity. Similar to a price relative, we
q 1
can define a quantity relative as Q 100
q 0
Various formulae for quantity index numbers are as given below :

q 1
1. Simple aggregative index Q 100
01
q
0
2. Simple average of quantity relatives
q
1
100
q 0 Q
(a) Taking A.M. Q 01
n n

logQ
(b) Taking G.M. Q 01 Antilog
n

210 LOVELY PROFESSIONAL UNIVERSITY