Page 216 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 10: Index Number

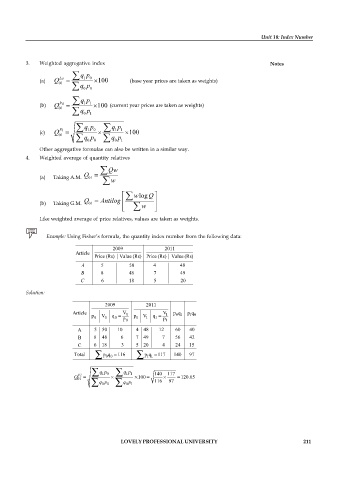
3. Weighted aggregative index Notes

q p 0
1
La
(a) Q 100 (base year prices are taken as weights)
01
q p
0 0
q p
(b) Q Pa 1 1 100 (current year prices are taken as weights)
01
q p
0 1
q p q p
(c) Q Fi 1 0 1 1 100
01
q p q p
0 0 0 1
Other aggregative formulae can also be written in a similar way.
4. Weighted average of quantity relatives

Qw
Q
(a) Taking A.M. 01
w
w logQ
Q Antilog
(b) Taking G.M. 01
w

Like weighted average of price relatives, values are taken as weights.

Example: Using Fisher’s formula, the quantity index number from the following data:

2009 2011
Article
Price (Rs) Value (Rs) Price (Rs) Value (Rs)
A 5 50 4 48
B 8 48 7 49
C 6 18 5 20

Solution:
2009 2011
Article V 0 V 1 p q p q
0 1
1 0
p 0 V 0 q 0 p 1 V 1 q 1
p
0 p 1

A 5 50 1 0 4 48 12 60 40
B 8 48 6 7 49 7 56 42
C 6 18 3 5 20 4 24 15
Total p q 116 p q 117 140 97
1 1
0 0
q p q p
Fi 1 0 1 1 140 117
Q 01 100 120.65
q p q p 116 97
0 1
0 0

LOVELY PROFESSIONAL UNIVERSITY 211