Page 225 - DMGT404 RESEARCH_METHODOLOGY

Unit 10: Time Series

3. To compare the pattern of seasonal variations of two or more time series in a given period Notes
or of the same series in different periods.
4. To eliminate the seasonal variations from the data. This process is known as
deseasonalisation of data.
The measurement of seasonal variation is done by isolating them from other components of a
time series. There are four methods commonly used for the measurement of seasonal variations.
These method are:
1. Method of Simple Averages

2. Ratio to Trend Method
3. Ratio to Moving Average Method
4. Method of Link Relatives

Notes In the discussion of the above methods, we shall often assume a multiplicative
model. However, with suitable modifications, these methods are also applicable to the
problems based on additive model.

10.6.1 Method of Simple Averages

This method is used when the time series variable consists of only the seasonal and random
components. The effect of taking average of data corresponding to the same period (say 1st
quarter of each year) is to eliminate the effect of random component and thus, the resulting
averages consist of only seasonal component. These averages are then converted into seasonal
indices, as explained in the following examples.

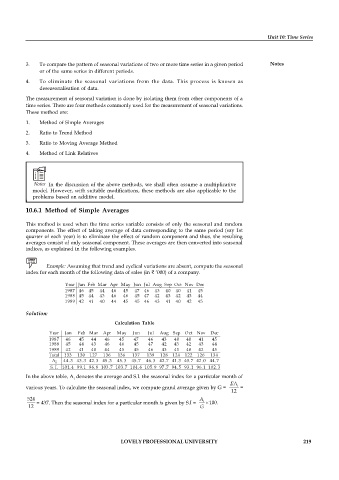
Example: Assuming that trend and cyclical variations are absent, compute the seasonal
index for each month of the following data of sales (in ‘000) of a company.

Year Jan Feb Mar Apr May Jun Jul Aug Sep Oct Nov Dec
1987 46 45 44 46 45 47 46 43 40 40 41 45
1988 45 44 43 46 46 45 47 42 43 42 43 44
1989 42 41 40 44 45 45 46 43 41 40 42 45
Solution:
Calculation Table

Year Jan Feb Mar Apr May Jun Jul Aug Sep Oct Nov Dec
1987 46 45 44 46 45 47 46 43 40 40 41 45
1988 45 44 43 46 46 45 47 42 43 42 43 44
1989 42 41 40 44 45 45 46 43 41 40 42 45
Total 133 130 127 136 136 137 139 128 124 122 126 134
A i 44.3 43.3 42.3 45.3 45. 3 45.7 46.3 42.7 41.3 40.7 42.0 44.7
S. I. 101. 4 99.1 96.8 103.7 103.7 104.6 105.9 97.7 94.5 93.1 96.1 102.3
In the above table, A denotes the average and S.I. the seasonal index for a particular month of
i
S A
various years. To calculate the seasonal index, we compute grand average given by G = i =
12
524 A
= 437. Then the seasonal index for a particular month is given by S.I = i  100.
12 G

LOVELY PROFESSIONAL UNIVERSITY 219