Page 166 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 8: Assignment Problem – Unbalanced

Solution: Notes
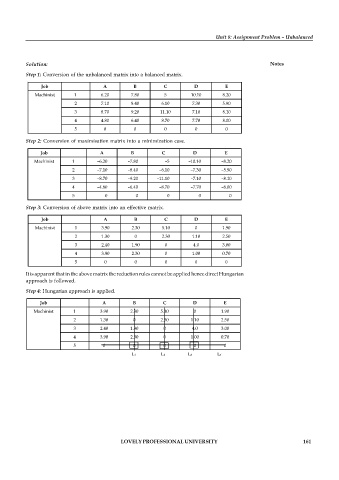
Step 1: Conversion of the unbalanced matrix into a balanced matrix.
Job A B C D E
Machinist 1 6.20 7.80 5 10.10 8.20
2 7.10 8.40 6.10 7.30 5.90
3 8.70 9.20 11.10 7.10 8.10
4 4.80 6.40 8.70 7.70 8.00
5 0 0 0 0 0

Step 2: Conversion of maximisation matrix into a minimization case.
Job A B C D E
Machinist 1 –6.20 –7.80 –5 –10.10 –8.20
2 –7.10 –8.40 –6.10 –7.30 –5.90
3 –8.70 –9.20 –11.10 –7.10 –8.10
4 –4.80 –6.40 –8.70 –7.70 –8.00
5 0 0 0 0 0

Step 3: Conversion of above matrix into an effective matrix.

Job A B C D E
Machinist 1 3.90 2.30 5.10 0 1.90
2 1.30 0 2.30 1.10 2.50
3 2.40 1.90 0 4.0 3.00
4 3.90 2.30 0 1.00 0.70
5 0 0 0 0 0

It is apparent that in the above matrix the reduction rules cannot be applied hence direct Hungarian
approach is followed.
Step 4: Hungarian approach is applied.

Job A B C D E
Machinist 1 3.90 2.30 5.10 0 1.90
2 1.30 0 2.30 1.10 2.50
3 2.40 1.90 0 4.0 3.00
4 3.90 2.30 0 1.00 0.70
5 0 0 0 0 0
L1 L2 L3 L4

LOVELY PROFESSIONAL UNIVERSITY 161