Page 185 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

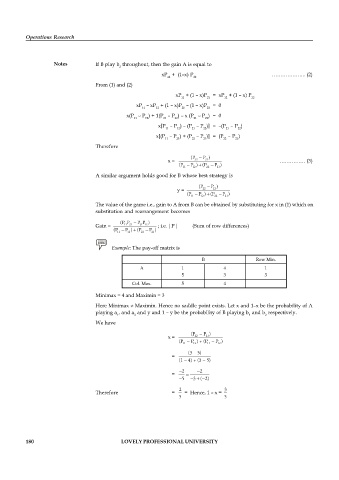
Notes If B play b throughout, then the gain A is equal to
2
xP + (1–x) P ……………….. (2)
12 22
From (1) and (2)
xP + (1 – x)P = xP + (1 – x) P
11 21 12 22
xP – xP + (1 – x)P – (1 – x)P = 0
11 12 21 22
x(P – P ) + 1(P – P ) – x (P – P ) = 0
11 12 21 22 21 22
x[P – P ) – (P – P )] = –(P – P )
11 12 21 22 21 22
x[(P – P ) + (P – P )] = (P – P )
11 21 22 21 22 21
Therefore

x = …………… (3)
  
A similar argument holds good for B whose best strategy is

y =
  
The value of the game i.e., gain to A from B can be obtained by substituting for x in (1) which on
substitution and rearrangement becomes

Gain = ; i.e. |P| (Sum of row differences)
  

Example: The pay-off matrix is
B Row Min.
A 1 4 1
5 3 3
Col. Max. 5 4

Minimax = 4 and Maximin = 3
Here Minimax  Maximin. Hence no saddle point exists. Let x and 1–x be the probability of A
playing a , and a and y and 1 – y be the probability of B playing b and b respectively.
1 2 1 2
We have

x =

=


= 
  

Therefore = = Hence, 1 – x =

180 LOVELY PROFESSIONAL UNIVERSITY