Page 95 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

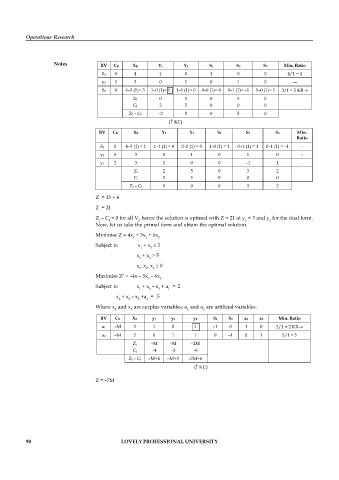
Notes BV CB XB Y1 Y2 S1 S2 S3 Min. Ratio
S1 0 4 1 0 1 0 0 4/1 = 4
y2 5 3 0 1 0 1 0 ––
S3 0 6–3 (1)= 3 1–0 (1)= 1 1–1 (1)= 0 0–0 (1)= 0 0–1 (1)= –1 1–0 (1)= 1 3/1 = 3 KR
ZJ 0 5 0 5 0
CJ 2 5 0 0 0
ZJ – CJ –2 0 0 5 0
( KC)

BV CB XB Y1 Y2 S1 S2 S3 Min.
Ratio
S1 0 4–3 (1) = 1 1–1 (1) = 0 0–0 (1) = 0 1–0 (1) = 1 0+1 (1) = 1 0–1 (1) = –1 -
y2 5 3 0 1 0 1 0 -
y1 2 3 1 0 0 –1 1 -
ZJ 2 5 0 3 2
CJ 2 5 0 0 0
ZJ – CJ 0 0 0 3 2

Z = 15 + 6
Z = 21
Z – C = 0 for all V, hence the solution is optimal with Z = 21 at y = 3 and y for the dual form.
J J j 1 2
Now, let us take the primal form and obtain the optimal solution.
Minimise Z = 4x + 3x + 6x
1 2 2
Subject to x + x  2
1 3
x + x  5
2 3
x , x , x  0
1 2 3
Maximise Z’ = –4x – 3x – 6x
2 3
Subject to x + x – x + a = 2
1 3 4 1
x + x – x +a = 5
2 3 5 2
Where x and x are surplus variables; a and a are artificial variables.
4 5 1 2
BV CB XB y1 y2 y3 S1 S2 a1 a2 Min. Ratio
a1 –M 2 1 0 1 –1 0 1 0 2/1 = 2 KR
a2 –M 5 0 1 1 0 –1 0 1 5/1 = 5
Zj –M –M –2M
Cj –4 –3 –6
Zj – Cj –M+4 –M+3 –2M+6
( KC)

Z = –7M

90 LOVELY PROFESSIONAL UNIVERSITY